
Contacts: Second-Order Elements (Part 3 of 3)
Table of contents
Here we go..
A quarter symmetric model of two blocks (see Figure 9) was used to highlight the problem. The top part was loaded with 0.25N and the bottom part was fixed at the base, the faces on the symmetry planes included symmetry boundary conditions and a frictionless, hard contact was applied between the two parts.
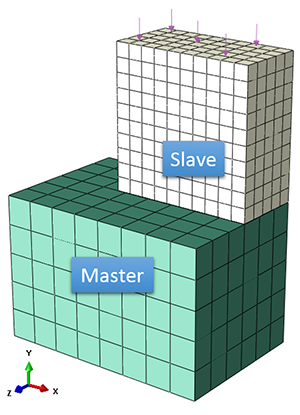
Figure 9: Example problem
Two analyses were conducted on this assembly in order to compare the behaviour of second-order and first-order element types in contact. In both analyses the master part element type was a first-order C3D8I. The element type of the slave part in the first-order analysis was also C3D8I, but in the second-order analysis this was changed to C3D20R.
Figure 10 highlights the CNORMF values of the slave surface for each analysis, in this case the node to surface discretization method was used.
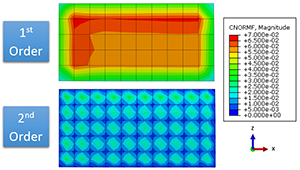
Figure 10: CNORMF on slave surface with node to surface discretization method
CNORMF is the total normal force at each node. Therefore, the magnitude increases as the number of elements a node is attached to increases. This helps to explain why the force drops at the edges of the part with the first-order slave surface, however it does not explain the tessellated results of the second-order contact.
At first glance, you would be forgiven for assuming that there is an error in the second-order model set-up, or you may perhaps try using the surface to surface discretization method to improve this odd behavior. So, let’s see what happens if you do change the discretization method:
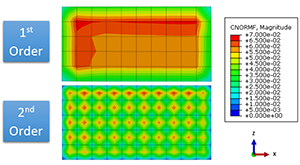
Figure 11: CNORMF on slave surface with surface to surface discretization method
Comparing
Figure 10 to Figure 11 it is apparent that the discretization method does affect the CNORMF values for the second-order elements, however, if you were to sum the CNORMF values you would find that in both cases it is equal to the total applied force (0.25N in this case), which is obviously correct.
So, which discretization method is preferable and why is this happening? Second order elements are often not well suited to contact when they are supporting the slave surface in a node to surface “hard” contact.
When applying a uniform pressure to a second-order element, the nodal forces represent equivalent forces, if the element does not include a mid-face node the force at the corner nodes act in the opposite direction to the pressure (see Figure 12).

Figure 12: Equivalent nodal forces produced by a uniform pressure, no mid-face node
Node to Surface Contacts
This is the behavior that is observed when using the surface-to-surface discretization method with C3D20(RH) elements. However, in the case of node to surface contacts, Abaqus adds a mid-face node to the slave surface elements (true for most 3D second-order elements) in order to negate the ambiguous nodal forces and the subsequent convergence issues that they may create. In the case of the C3D20(RH), an additional 7 nodes are added (6 mid-face and 1 mid-element) to create a C3D27(RH) element. Figure 13 shows the CNORMF vectors for the C3D27R element type.

Figure 13: Equivalent nodal forces produced by a uniform pressure, with mid-face nodes
This explains the behavior seen in Figure 10 and Figure 11 but, ultimately, the question is whether it is a problem or not? The answer depends on your model and required outputs, but in general consider the following:
- Try to use first order element types at contacts (especially at the slave surface). This helps to reduce solve time and increases the accuracy of nodal force values.
- If you have to use second-order elements in the contact region then try to use surface to surface discretization to prevent Abaqus form creating additional mid-face nodes, thus reducing solve time.
- Consider whether or not the CNORMF behavior highlighted in this blog is a problem.
- If you do not require nodal force values then the behavior may be acceptable as it will not necessarily have a significant effect on other outputs e.g. stress and pressure.
Learn more about Abaqus.


